"A very small cause which escapes our notice determines a considerable effect that we cannot fail to see, and then we say the effect is due to chance. If we knew exactly the laws of nature and the situation of the universe at the initial moment, we could predict exactly the situation of that same universe at a succeeding moment. But even if it were the case that the natural laws had no longer any secret for us, we could still only know the initial situation *approximately*. If that enabled us to predict the succeeding situation with *the same approximation*, that is all we require, and we should say that the phenomenon had been predicted, that it is governed by laws. But it is not always so; it may happen that small differences in the initial conditions produce very great ones in the final phenomena. A small error in the former will produce an enormous error in the latter. Prediction becomes impossible, and we have the fortuitous phenomenon"
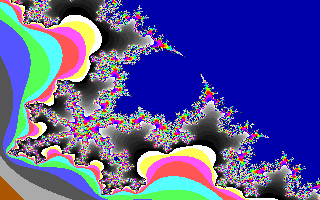
Basically, chaos theory is the study of non-linear dynamics, or turbulence. Such effects occur in systems like a flag fluttering in the wind, water flowing in a stream or weather systems in the atmosphere. Other examples include water dripping from a tap, smoke swirling in the air or air flowing over the wing of a plane. The mathematical representations of the change from order to disorder are called fractals. They have the property of self-similarity on all scales. They are generated by iterating simple equations and plotting the output on a computer screen.
Traditional education in the dynamics of physical systems never revealed the potential of such complexity, because traditional education focused on systems that were linear. In these linear systems, the laws of proportionality rule. The more force you apply, the faster you go. The faster you go, the farther you travel. However, in the real world, most systems are dynamical and nonlinear. They contain irregularities that do not vary neatly as functions of the other variables. These very small differences gave the systems sensitive dependence on initial conditions and made for messy and difficult calculations. This wreaked havoc with classical deterministic mechanics. No one, however, seemed to think of nonlinearity as a creative force. Yet it is nonlinearity that creates the beautiful patterns of fractal geometry.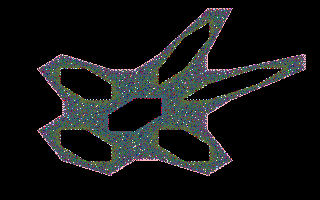
All of the fractals on this page were made with a program called FRACTINT, which is widely available for free on the web and on most online services such as CIS. This figure is often called Gingerbread Man and is generated with the simple equation XN = 1 - Y + ABS (X)There are lots of nice fractal pages, but you might start at Ferry's Fractal Pictures. Other nice sites exist by Ken Musgrave, Art Baker and Sharon Webb.